Math12: Chapter 8- Exponential and its inverse lnx function

Warm up : Logarithmic Rules

How to sketch reflection in exponential graphs

How to sketch stretch in exponential graphs

Solve for x,

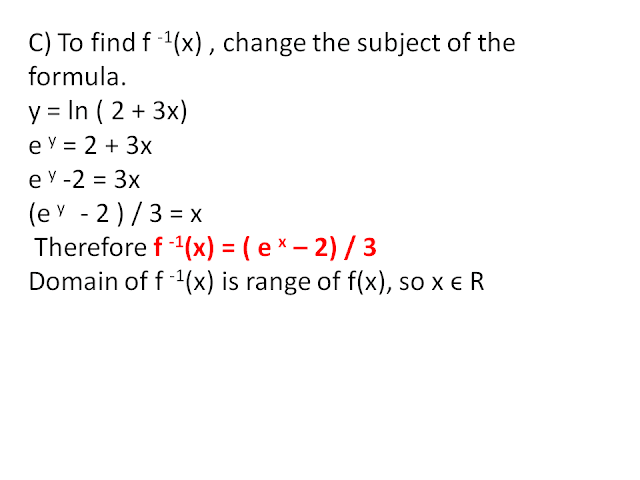
Solving logarithmic equations:

Solve log₂(x) = 4.

Since this is "log equals a number", rather than "log equals log", we can solve by using The Relationship:

log₂(x) = 4
2⁴ = x 16 = x

Solve log₂(8) = x.

I can solve this by converting the logarithmic statement into its equivalent exponential form, using The Relationship:

log₂(8) = x 2^x = 8

But 8 = 2³, so:

2^x = 2³ x = 3

Solve log₂(x) + log₂(x – 2) = 3

We can't do anything yet, because we don't yet have "log equals a number". So we'll need to use log rules to combine the two terms on the left-hand side of the equation:

log₂(x) + log₂(x – 2) = 3
log₂((x)(x – 2)) = 3
log₂(x² – 2x) = 3

Then we'll use The Relationship to convert the log form to the corresponding exponential form, and then we'll solve the result:

log₂(x² – 2x) = 3
2³ = x² – 2x
8 = x² – 2x
0 = x² – 2x – 8
0 = (x – 4)(x + 2)
x = 4, –2 But if x = –2, then "log₂(x)", from the original logarithmic equation, will have a negative number for its argument (as will the term "log₂(x – 2)"). Since logs cannot have zero or negative arguments, then the solution to the original equation cannot be x = –2.
The solution is x = 4.